Alteryx Designer Desktop Discussions
Find answers, ask questions, and share expertise about Alteryx Designer Desktop and Intelligence Suite.- Community
- :
- Community
- :
- Participate
- :
- Discussions
- :
- Designer Desktop
- :
- Lift Curve
Lift Curve
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
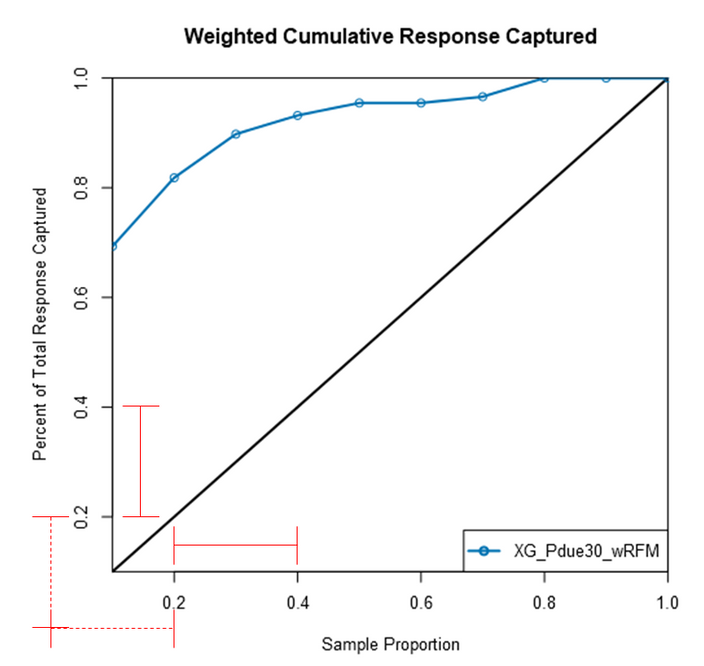
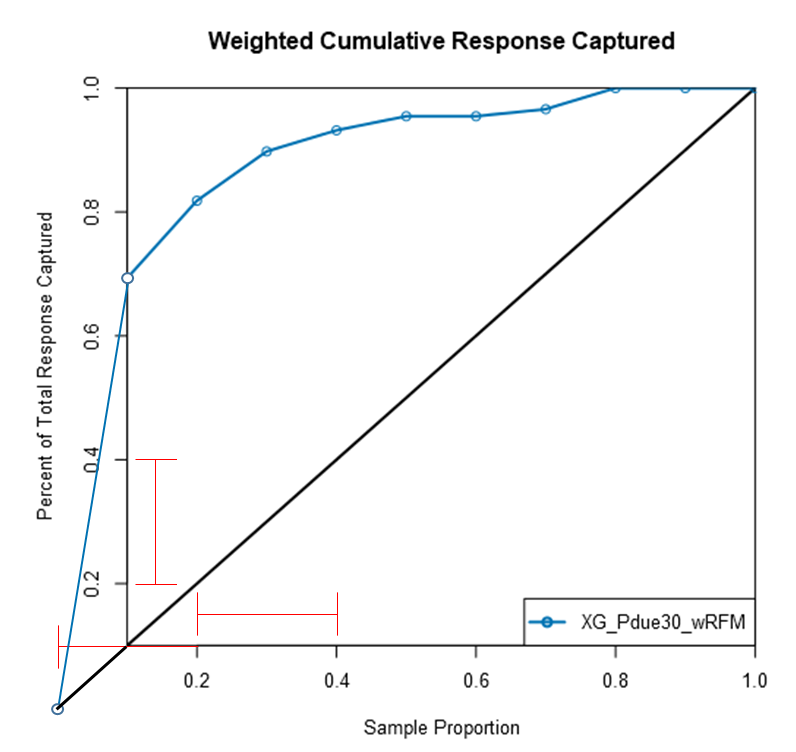
Here is the lift chart output, the chart starts at (0.1,0.1) not at the origin of (0,0) is there a reason to that?
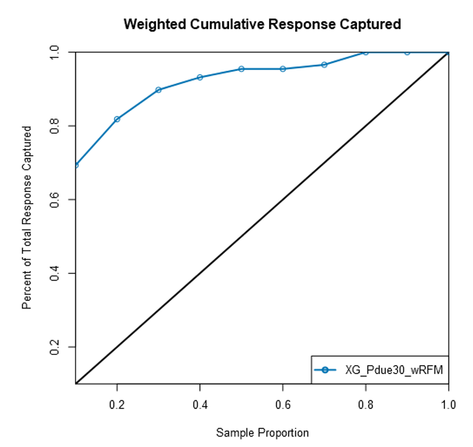
Here are some scaling on both X and Y axis...
If we put eh point to the origin and redraw the cumulative response, is this a more accurate graph?
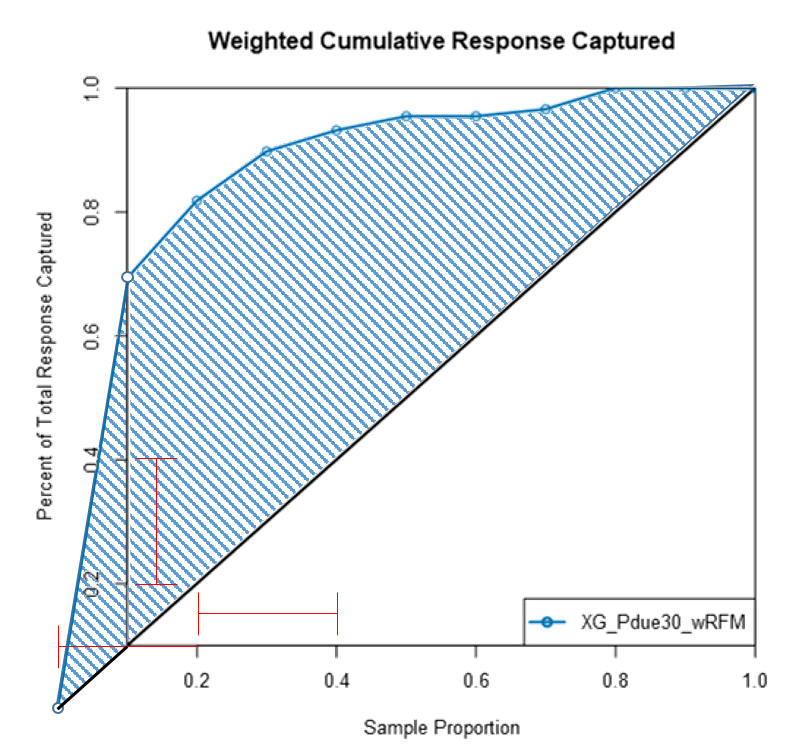
The area under the curve (AUC or ROC metric) does it include the drawn area as well or not?
Best
Solved! Go to Solution.
- Labels:
-
Predictive Analysis
-
Reporting
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This question is old and I am not with Alteryx support, but I was doing something related. I thought I would comment.
I believe the AUC reported does indeed include the area between 0 and 0.1 even though it is not shown.
I checked this using the gains chart that is also provide in the output. So say Alteryx gives you a gains chart like this:
| Decile | Cum_Pct |
| 0 | g0 |
| 10 | g1 |
| 20 | g2 |
| 30 | g3 |
| 40 | g4 |
| 50 | g5 |
| 60 | g6 |
| 70 | g7 |
| 80 | g8 |
| 90 | g9 |
| 100 | g10 |
g0-g10 are numbers between 0 and 1.
The x-coordinates of the points in the curve are Decile / 100. The y-coordinates of points in the curve are g0 - g10 (though point (0,g0) is omitted as you pointed out.
Using the trapezoidal rule, the AUC including the area that's 'falling off the picture' is like this:
AUC ~= sum(i= 1 to i=10) {((Decile[i] - Decile[i-1])/100)*(g[i] + g[i-1])/2}
It may look a little funky, but this calculation is easy to do in Excel. Doing that in my own example, I can match the AUC Alteryx is outputting to 3 decimal places. So I think it's the case that they're including the 'falling of the chart' area. Why they didn't start the chart at 0 for the picture, I don't know. I speculate that the incremental option would logically start at 0.1 and they decided to start both versions of the chart at the same place.
Best,
Jose
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Oh noticed one other thing. You mentioned ROC in your question and this is not an ROC curve. The AUC reported by the Lift tool is the AUC under the Gains curve. I think there are other Alteryx tools that calculate AUC for the ROC curve but I haven't used them.
-
Academy
5 -
ADAPT
2 -
Adobe
201 -
Advent of Code
2 -
Alias Manager
76 -
Alteryx Copilot
21 -
Alteryx Designer
7 -
Alteryx Editions
59 -
Alteryx Practice
19 -
Amazon S3
148 -
AMP Engine
247 -
Announcement
1 -
API
1,202 -
App Builder
113 -
Apps
1,356 -
Assets | Wealth Management
1 -
Basic Creator
10 -
Batch Macro
1,531 -
Behavior Analysis
244 -
Best Practices
2,678 -
Bug
713 -
Bugs & Issues
1 -
Calgary
67 -
CASS
53 -
Chained App
265 -
Common Use Cases
3,804 -
Community
24 -
Computer Vision
83 -
Connectors
1,413 -
Conversation Starter
3 -
COVID-19
1 -
Custom Formula Function
1 -
Custom Tools
1,932 -
Data
1 -
Data Challenge
9 -
Data Investigation
3,472 -
Data Science
2 -
Database Connection
2,202 -
Datasets
5,191 -
Date Time
3,219 -
Demographic Analysis
184 -
Designer Cloud
725 -
Developer
4,335 -
Developer Tools
3,508 -
Documentation
524 -
Download
1,026 -
Dynamic Processing
2,917 -
Email
924 -
Engine
145 -
Error Message
2,235 -
Events
194 -
Expression
1,862 -
Financial Services
1 -
Full Creator
1 -
Fun
2 -
Fuzzy Match
708 -
Gallery
658 -
GenAI Tools
1 -
General
1 -
Google Analytics
156 -
Help
4,689 -
In Database
962 -
Input
4,267 -
Installation
352 -
Interface Tools
1,891 -
Iterative Macro
1,086 -
Join
1,945 -
Licensing
245 -
Location Optimizer
61 -
Machine Learning
257 -
Macros
2,838 -
Marketo
12 -
Marketplace
22 -
MongoDB
83 -
Off-Topic
4 -
Optimization
746 -
Output
5,217 -
Parse
2,316 -
Power BI
224 -
Predictive Analysis
934 -
Preparation
5,137 -
Prescriptive Analytics
205 -
Professional (Edition)
2 -
Publish
256 -
Python
849 -
Qlik
39 -
Question
1 -
Questions
2 -
R Tool
477 -
Regex
2,333 -
Reporting
2,426 -
Resource
1 -
Run Command
569 -
Salesforce
276 -
Scheduler
410 -
Search Feedback
3 -
Server
619 -
Settings
929 -
Setup & Configuration
3 -
Sharepoint
615 -
Spatial Analysis
596 -
Tableau
511 -
Tax & Audit
1 -
Text Mining
465 -
Thursday Thought
4 -
Time Series
429 -
Tips and Tricks
4,166 -
Topic of Interest
1,120 -
Transformation
3,699 -
Twitter
23 -
Udacity
84 -
Updates
1 -
Viewer
2 -
Workflow
9,901
- « Previous
- Next »
| User | Count |
|---|---|
| 106 | |
| 82 | |
| 70 | |
| 54 | |
| 40 |