How precise are latitude and longitude

[Ayshdr99]

Suppose I am given latitudes and longitudes of some points in a tabular format. How precise are these points?

Are these points truly dimensionless so that the point does not have any length or width? My confusion arose because if I know the latitude and longitude of a Walmart store in a given place, is it possible that the four corners of the store have four different latitudes and longitudes?

Thanks

[echuong1]

Latitude and longitude are usually represented by a location's geographic center.

[CharlieS · Accepted solution]

HI @Ayshdr99 

Yes they are "dimensionless" like you described. Using a single point to define a Walmart is to imply that the Walmart is dimensionless. That single point could be 1) an approximate center of the building area, 2) the front doors/access point, 3) the street intersection/"curb cut" (common result from geocoding), 4) any random point on the property, or 5) inaccurate and not be a Walmart at all. 

Depending on the purpose of your analysis, a point on the property may be sufficient, but other use cases might require a specific point (like 1-3 above), or a polygon-type spatial object to represent the building or property area, 

Additional notes on point accuracy:

The decimal degrees are generally representative of accuracy of that singular point:

https://en.wikipedia.org/wiki/Decimal_degrees

- The accuracy conversation is entirely dependent on the coordinate system/datum. WGS84 is the default for 99.999% of applications (Alteryx included), but there are alternative coordinate systems available for specialized purposes. Plotting water lines for a municipality? There are localized coordinate systems that could be far more accurate, but would only apply to that local area.

- Just because a measurement has n digits doesn't mean it's actually measured that accurately. Always investigate sources.

- Elevation is ignored here. 

[Hollingsworth]

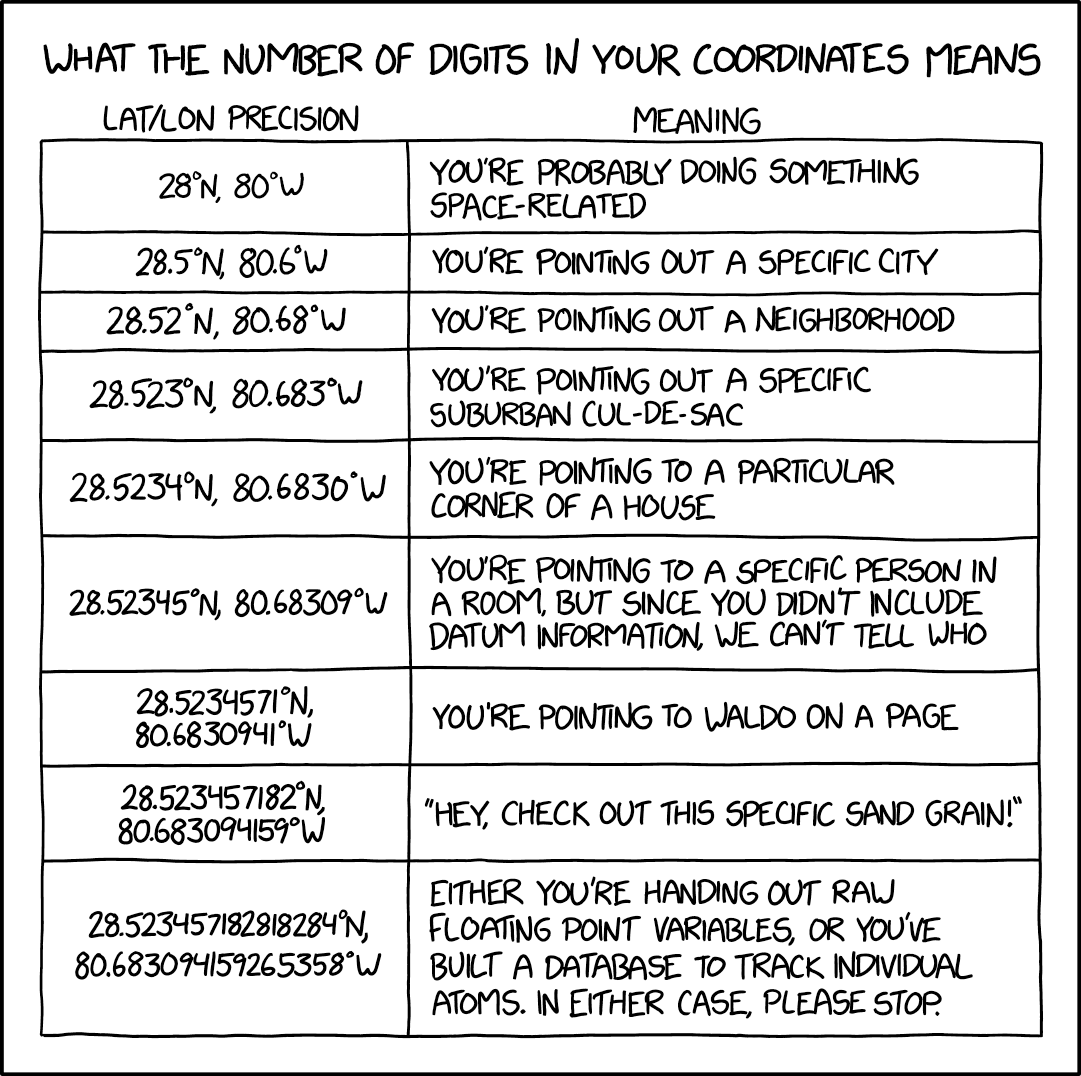
This post got me thinking about the question, "How many significant digits do I need in my latitude/longitude values?"

The short answer is that five significant digits with get you to within five feet of the exact location.

The proof for this is contained in the attached workflow.

[Hollingsworth]

As always, XKCD has the last word.

https://xkcd.com/2170/