Weekly Challenges
Solve the challenge, share your solution and summit the ranks of our Community!Also available in | Français | Português | Español | 日本語
IDEAS WANTED
Want to get involved? We're always looking for ideas and content for Weekly Challenges.
SUBMIT YOUR IDEA- Community
- :
- Community
- :
- Learn
- :
- Academy
- :
- Challenges & Quests
- :
- Weekly Challenges
- :
- Challenge #135: Locker Problem
Challenge #135: Locker Problem
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you for participating in the Grand Prix challenges last week!
Next week's challenge will be posted during @NicoleJohnson's Inspire Europe Weekly Challenge (10:30AM on Wednesday 10/10)! Finally, those on GMT challengers will finally have first crack at the challenge. Unless @patrick_digan wakes up at 5:30AM Eastern.
Onto this week's challenge!
There are 1000 lockers in a high school with 1000 students. The problem begins with the first student opening all 1000 lockers; the second student closes lockers 2,4,6,8,10 and so on to locker 1000; the third student changes the state (opens lockers closed, closes lockers open) on lockers 3,6,9,12,15 and so on; the fourth student changes the state of lockers 4,8,12,16 and so on. This goes on until
every student has had a turn.
When all 1,000 students have finished, which locker doors are open?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Back to school! My solution :)
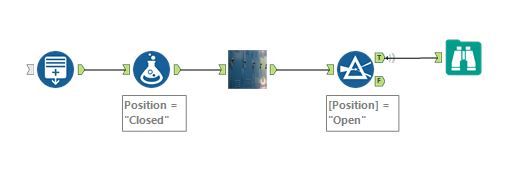 Workflow - Fun using the Generate Rows tool without an input, rarely have had opportunities to do that.
Workflow - Fun using the Generate Rows tool without an input, rarely have had opportunities to do that. Locker Macro, using a Floor formula to determine which lockers to open/close!
Locker Macro, using a Floor formula to determine which lockers to open/close!Cheers,
NJ
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Kind of messy
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I've got my alarm set for 5:30 am next wednesday!
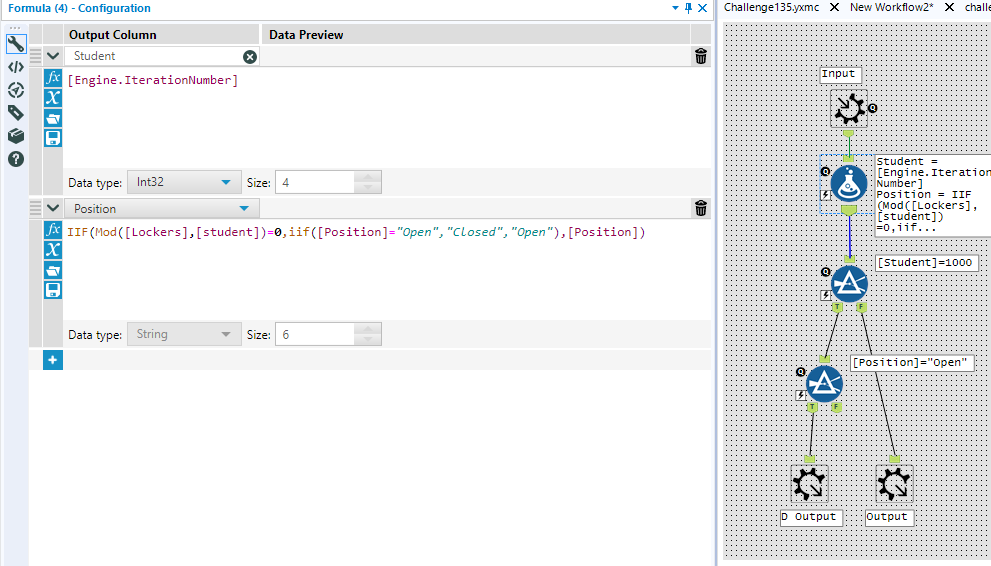
There are some really creative solutions when @Ned posted this problem a few years ago.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I'm imagining this is a very loud exercise!
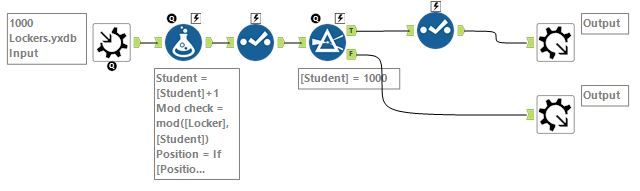
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fun challenge.
Ok, all you Math gurus. Can anyone explain why, iteratively applying the sieve of Eratosthenes, should generate that particular, very familiar, sequence of numbers? Is all of math beautifully connected at a level just below what common folk like me can see?
And what's up with Euler's Identity anyway
Dan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Solution attached.
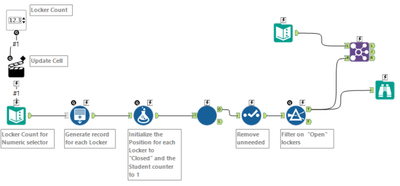
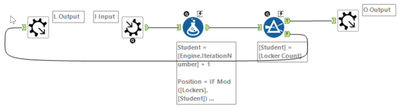
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
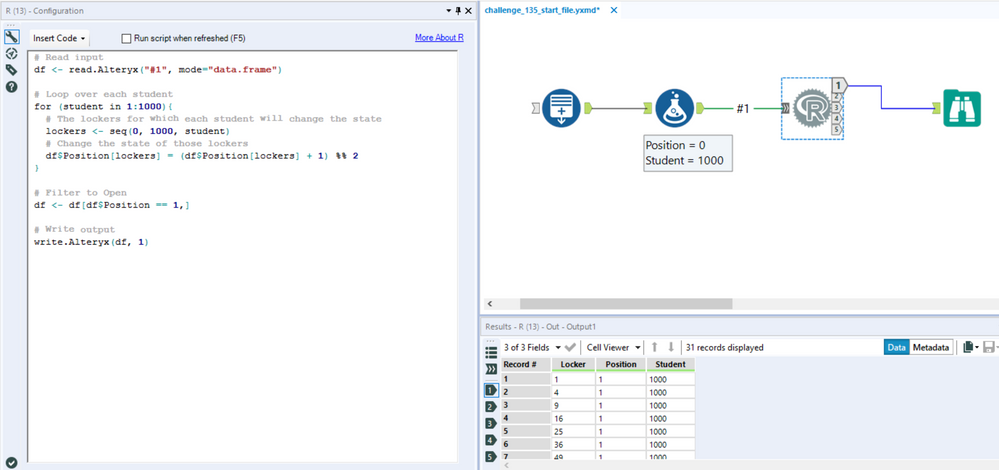 Workflow
Workflow-
Advanced
299 -
Apps
27 -
Basic
156 -
Calgary
1 -
Core
155 -
Data Analysis
186 -
Data Cleansing
6 -
Data Investigation
7 -
Data Parsing
15 -
Data Preparation
234 -
Developer
35 -
Difficult
85 -
Expert
16 -
Foundation
13 -
Interface
39 -
Intermediate
266 -
Join
211 -
Macros
61 -
Parse
141 -
Predictive
20 -
Predictive Analysis
14 -
Preparation
272 -
Reporting
55 -
Reporting and Visualization
17 -
Spatial
60 -
Spatial Analysis
53 -
Time Series
1 -
Transform
225
- « Previous
- Next »



