Alteryx Designer Desktop Discussions
Find answers, ask questions, and share expertise about Alteryx Designer Desktop and Intelligence Suite.- Community
- :
- Community
- :
- Participate
- :
- Discussions
- :
- Designer Desktop
- :
- greatest common divisor
greatest common divisor
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Dear Alteryx expert,
I am looking for an easy way to calculate the GCD of a string of numbers. To put my request in context, I have joined an excel spreadsheet where for two materials I have the volumes historically ordered. I would then like to generate per material what is the GCD of volumes to better understand whether we can see a pattern/ lot sizes.
Many thanks!
- Labels:
-
Preparation
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This is a very interesting one.
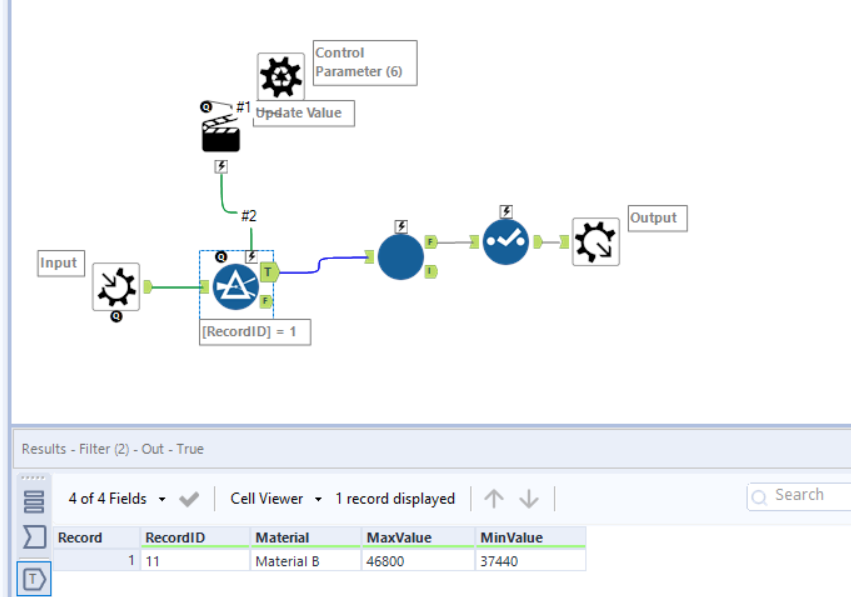
I use a Batch Macro and an iterative macro and I think I did it.
If you agree, can I submit this idea for a weekly challenge?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Qiu
I fully agree to your submission idea
Thx
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi @Jean-Remi
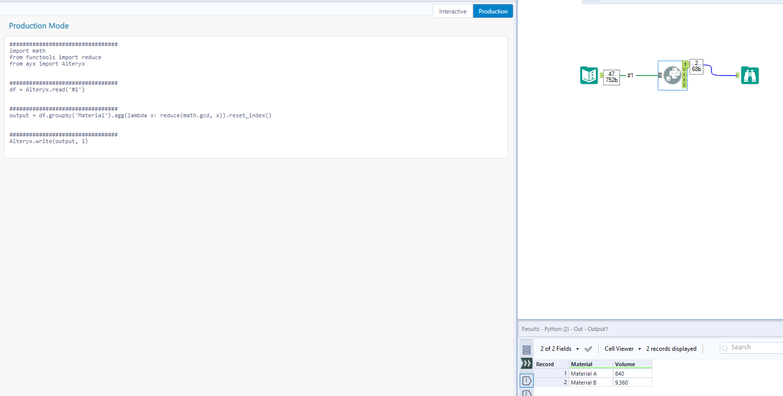
This is a really fun challenge, and I wanted to see if one could do it without macros or code.
My idea was to first generate all of the divisors, and then figure out a way to figure out which were common to all of the numbers, and finally the greatest of those.
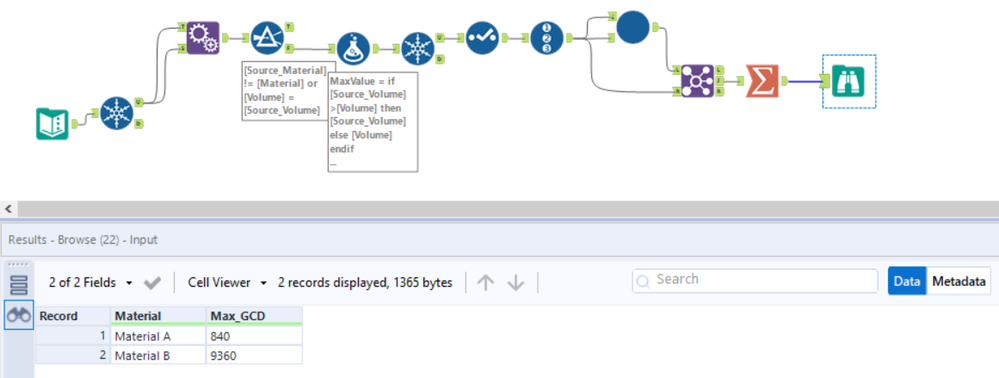
So, first step was to calculate how many different records are for each material. If a divisor were common to all of those records, the counts would match (e.g., for the 34 records for material A, a divisor could only be common if it counts to 34 when matching up to the numbers)
Next, generate all possible numbers that can be divisors by simply generating rows for each record, from 2 up until the value of half the record.
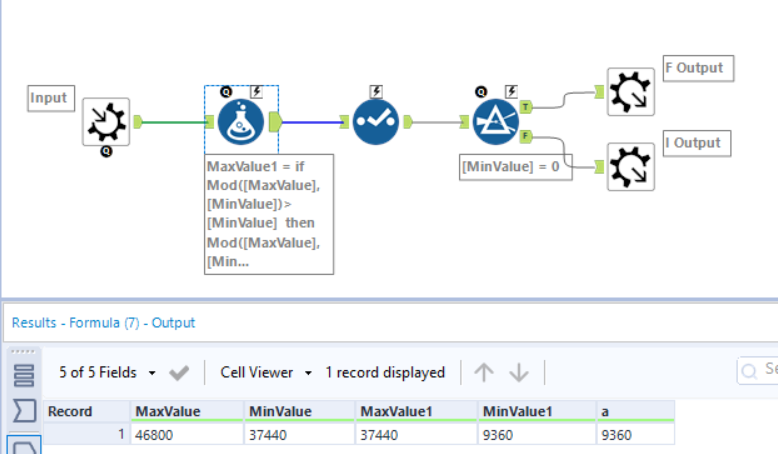
Mod is a great function to determine if a number is a divisor. If the modulo of 2 numbers is a zero, then yes, otherwise no. Filter out all of the numbers that aren't divisors.
Match up the records to see common divisors, and then summarize how many times each of these numbers appear. If they appear the same number of times as records in the material, then they are common.
Another summary tool finds the max of these numbers per material. you could also sort and then use the sample tool to pull the first (or last) record per material.
Let me know if that helps
Cheers!
Esther
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Excellent solutions all!
I just like to propose a small optimization to @estherb47's solution. You only have to generate rows up to the square root of the number as opposed to 1/2 the number. Since sqrt(x)*sqrt(x) = x, you know that anything larger than sqrt(x) has to be multiplied by a number smaller than sqrt(x).
Dan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
-
Academy
5 -
ADAPT
2 -
Adobe
201 -
Advent of Code
2 -
Alias Manager
76 -
Alteryx Copilot
20 -
Alteryx Designer
7 -
Alteryx Editions
58 -
Alteryx Practice
19 -
Amazon S3
147 -
AMP Engine
246 -
Announcement
1 -
API
1,198 -
App Builder
113 -
Apps
1,354 -
Assets | Wealth Management
1 -
Basic Creator
10 -
Batch Macro
1,527 -
Behavior Analysis
244 -
Best Practices
2,676 -
Bug
713 -
Bugs & Issues
1 -
Calgary
67 -
CASS
53 -
Chained App
265 -
Common Use Cases
3,797 -
Community
24 -
Computer Vision
82 -
Connectors
1,411 -
Conversation Starter
3 -
COVID-19
1 -
Custom Formula Function
1 -
Custom Tools
1,931 -
Data
1 -
Data Challenge
9 -
Data Investigation
3,468 -
Data Science
2 -
Database Connection
2,199 -
Datasets
5,187 -
Date Time
3,216 -
Demographic Analysis
184 -
Designer Cloud
723 -
Developer
4,333 -
Developer Tools
3,506 -
Documentation
523 -
Download
1,024 -
Dynamic Processing
2,913 -
Email
922 -
Engine
145 -
Error Message
2,234 -
Events
193 -
Expression
1,862 -
Financial Services
1 -
Full Creator
1 -
Fun
2 -
Fuzzy Match
707 -
Gallery
657 -
GenAI Tools
1 -
General
1 -
Google Analytics
156 -
Help
4,681 -
In Database
961 -
Input
4,263 -
Installation
352 -
Interface Tools
1,889 -
Iterative Macro
1,082 -
Join
1,944 -
Licensing
243 -
Location Optimizer
61 -
Machine Learning
257 -
Macros
2,835 -
Marketo
12 -
Marketplace
22 -
MongoDB
83 -
Off-Topic
4 -
Optimization
745 -
Output
5,211 -
Parse
2,314 -
Power BI
224 -
Predictive Analysis
934 -
Preparation
5,133 -
Prescriptive Analytics
205 -
Professional (Edition)
2 -
Publish
256 -
Python
847 -
Qlik
39 -
Question
1 -
Questions
1 -
R Tool
477 -
Regex
2,330 -
Reporting
2,421 -
Resource
1 -
Run Command
567 -
Salesforce
276 -
Scheduler
410 -
Search Feedback
3 -
Server
619 -
Settings
929 -
Setup & Configuration
3 -
Sharepoint
614 -
Spatial Analysis
595 -
Tableau
511 -
Tax & Audit
1 -
Text Mining
465 -
Thursday Thought
4 -
Time Series
428 -
Tips and Tricks
4,163 -
Topic of Interest
1,119 -
Transformation
3,694 -
Twitter
23 -
Udacity
84 -
Updates
1 -
Viewer
2 -
Workflow
9,887
- « Previous
- Next »