Challenge #168: Dice Game - Born to Solve

[rinacio]

:)

[PhilipMannering]

First time using the Frequency Table tool. Solution attached.

Spoiler

Workflow

[T_Willins]

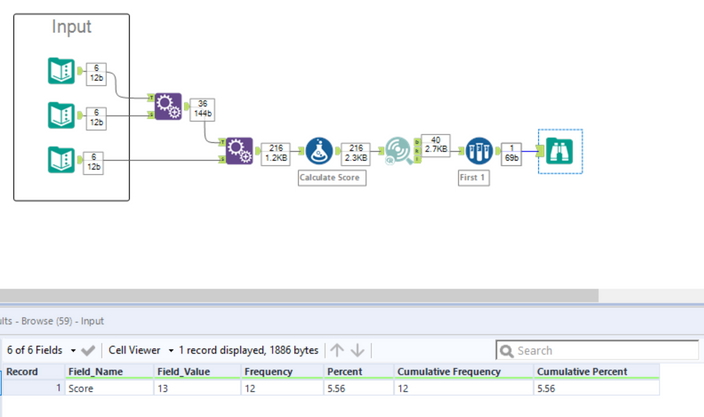
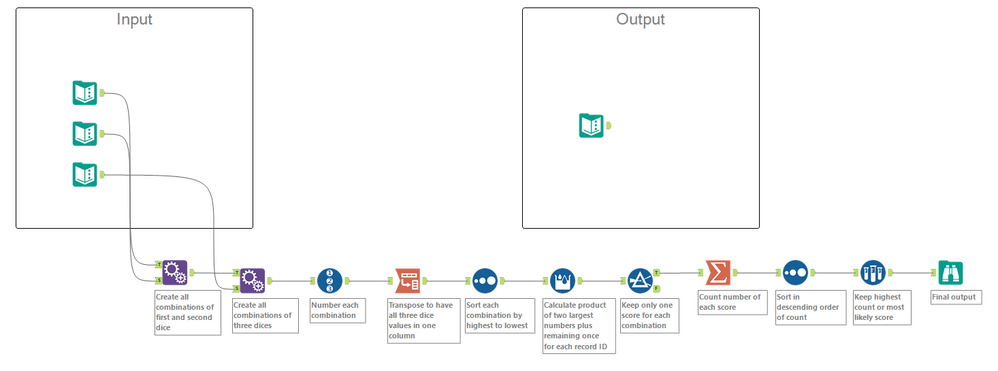
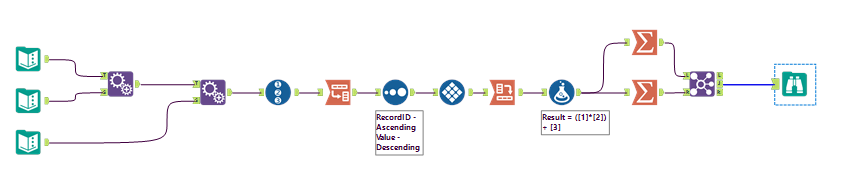
Hi @JORGE4900.  I now understand your point and I had to think about this and algebraic theory of probability.  Really this is two separate events:  The dice roll and the math.  Since each die is independent of each other, the algebraic theory of probability for any given roll is 6^3.  After the roll we then apply the math rules to get our roll total.  Thus, while there are 56 unique possible math combinations (thank you unique tool!), there are 216 possible rolls that need to be evaluated.

[kelly_gilbert]

Full disclosure: I attended this session at Inspire. It was fun to see my fellow weekly challengers, @estherb47 and @patrick_digan, live (and we learned how to properly pronounce @patrick_digan's name!) Since I lead a weekly challenge meetup at our office each week, I wanted to see how others articulate their thought process while solving (which @estherb47 demonstrated very well). 

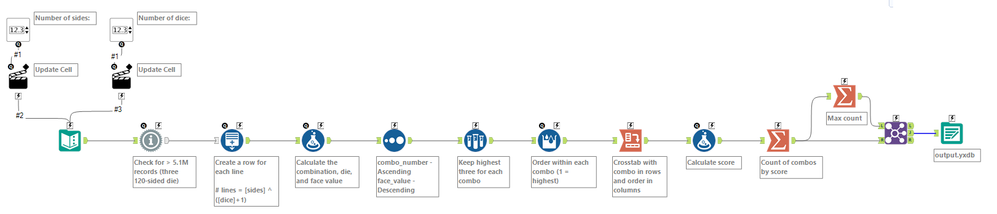
Since I'd already seen the "normal" solution, I tried to make this one dynamic (number of dice, number of sides).

As an aside, the largest number of sides available on a die is 120 ("the ultimate fair dice allowed by Mother Nature").

As another aside, with three 120-sided die, there are 136 different scores tied with a count of 183, and those scores range from 3,137 to 4,231!

Spoiler

[EmilyCR]

Would have been great to see this one live! Fun challenge :)

[diksha1107]

Incredible use of Mean, Mode, Median!

Solution attached!

[JoshuaGostick]

Fun challenge!

Spoiler

[Hannah_Lissaman]

Nice challenge! Here's a quick solution (although I'm sure it could be done more elegantly)

Spoiler

[john_miller9]

Really good challenge that gets you thinking.  Can't wait to review how others approached this and solved!

Spoiler